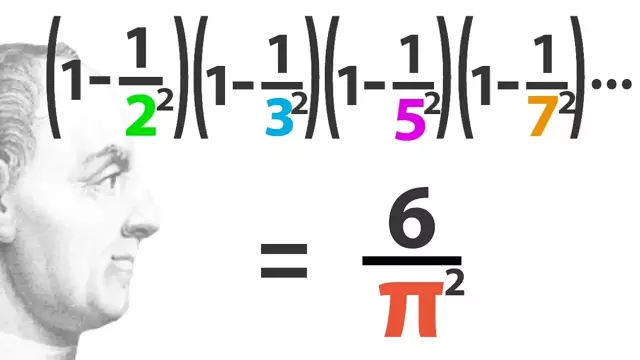2017-09-08

[public] 263K views, 10.6K likes, 94.0 dislikes audio only

Mathologer

NEW (Christmas 2019). Two ways to support Mathologer

Mathologer Patreon: https://www.patreon.com/mathologer

Mathologer PayPal: paypal.me/mathologer

(see the Patreon page for details)

What has pi to do with the prime numbers, how can you calculate pi from the licence plate numbers you encounter on your way to work, and what does all this have to do with Riemann's zeta function and the most important unsolved problem in math? Well, Euler knew most of the answers, long before Riemann was born.

I got this week's pi t-shirt from here: https://shirt.woot.com/offers/beautiful-pi

As usual thank you very much to Marty and Danil for their feedback on an earlier version of this video and Michael (Franklin) for his help with recording this video..

Here are a few interesting references to check out if you can handle more maths: J.E. Nymann, On the probability that k positive integers are relatively prime, Journal of number theory 4, 469--473 (1972) http://www.sciencedirect.com/science/article/pii/0022314X72900388 (contains a link to a pdf file of the article).

Enjoy :)