2016-11-25

[public] 213K views, 9.35K likes, 46.0 dislikes audio only

3Blue1Brown

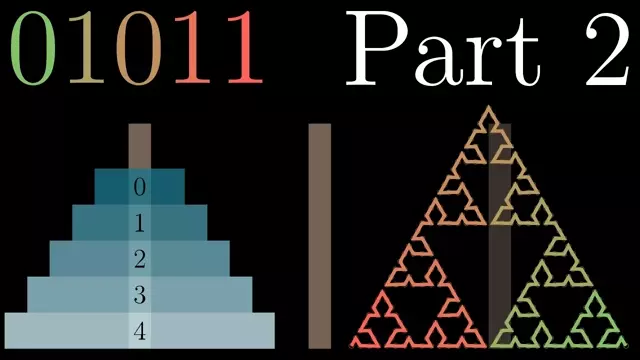
After seeing how binary counting can solve the towers of Hanoi puzzle in the last video, here we see how ternary counting solve a constrained version of the puzzle, and how this gives a way to walk through a Sierpinski triangle graph structure.

Thanks to Desmos for their help in supporting this video. They're hiring, and anyone interested should check out https://www.desmos.com/careers

Thanks to all Patreon supporters as well, you can support and get early access to future "Essence of" series here: https://www.patreon.com/3blue1brown

I also want to give a special shoutout to the following patrons: CrypticSwarm, Ali Yahya, Dave Nicponski, Juan Batiz-Benet, Yu Jun, Othman Alikhan, Markus Persson, Joseph John Cox, Luc Ritchie, Einar Wikheim Johansen, Rish Kundalia, Achille Brighton, Kirk Werklund, Ripta Pasay, Felipe Diniz, Chris, Curtis Mitchell, Ari Royce, Bright , Myles Buckley, Robert P Zuckett, Andy Petsch, Otavio good, Karthik T, Steve Muench, Viesulas Sliupas, Steffen Persch, Brendan Shah, Andrew Mcnab, Matt Parlmer, Naoki Orai, Dan Davison, Jose Oscar Mur-Miranda, Aidan Boneham, Brent Kennedy, Henry Reich, Sean Bibby, Paul Constantine, Justin Clark, Mohannad Elhamod, Denis, Ben Granger, Jeffrey Herman, Jacob Young.